Warning.
Enemy scenario:
|
|
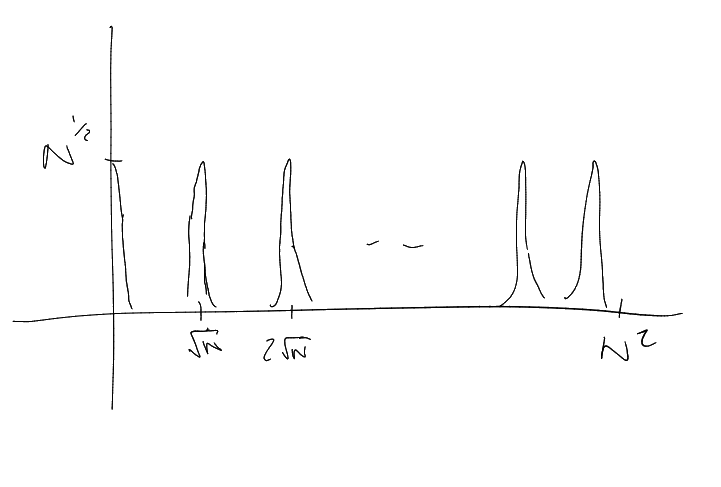
We can calculate that the above expression is in fact
Correction for lecture 2: Number Theory Lemma.
True statements:
|
|
and
See Terence Tao notes online.
Explaining
We will be using
Question:
|
|
For example,
Recall:
Reasonable conjecture?
Yes, reasonable.
Khinchin’s inequality: May select
|
|
Constant integral:
|
|
Warning.
Enemy scenario:
|
|

We can calculate that the above expression is in fact
It turns out that this is (roughly speaking) the only problem.
Why do we care?
|
|
|
|
Decoupling doesn’t know how to take advantage of
|
|
Facts:
If
Plancherel’s Theorem:
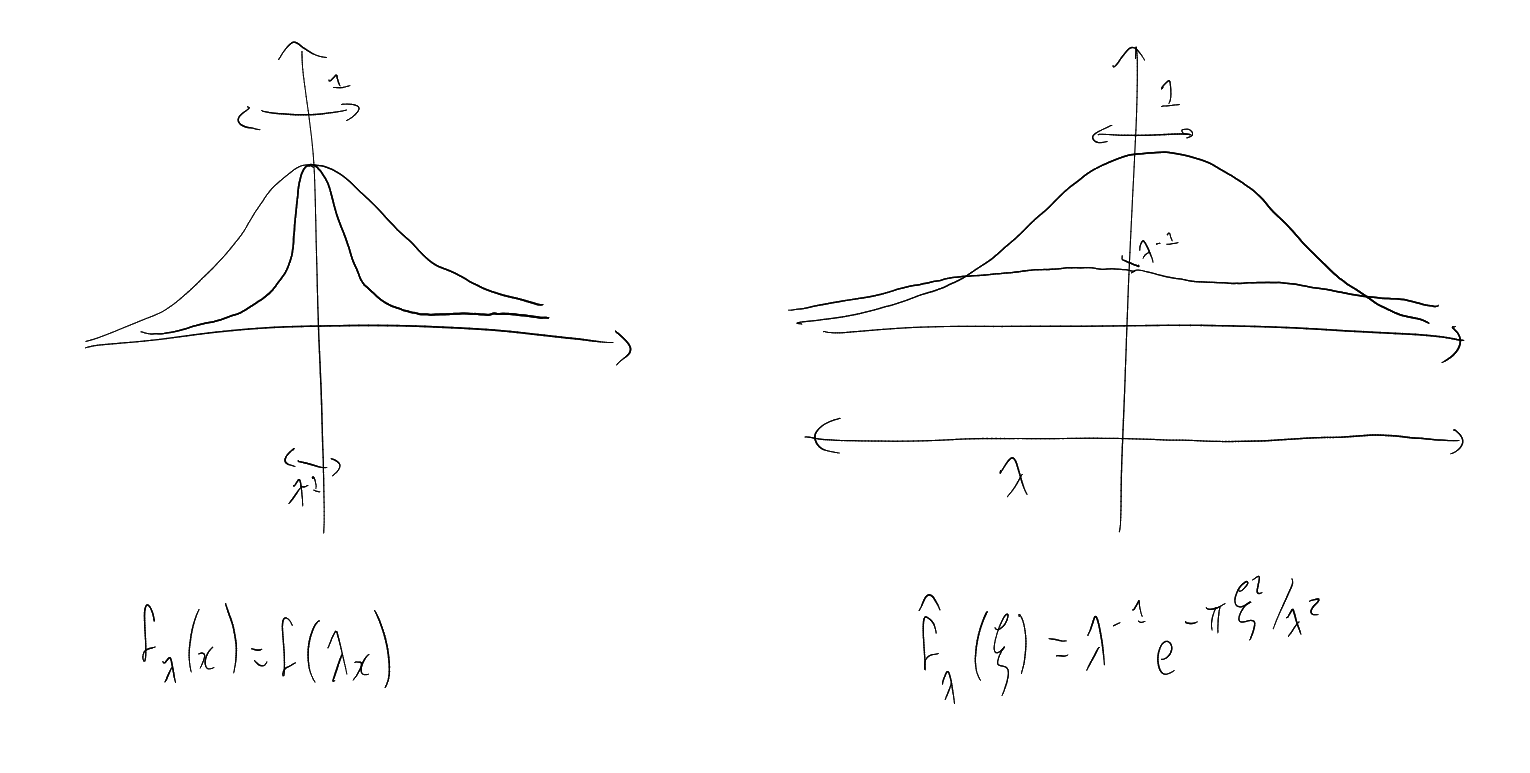
On the left: mass of
There is a general formula for
Translations are dual to modulations:
Basic question about
Plancherel’s:
|
|
(isometry on
|
|
(contraction from
By interpolation (Marcinkiewicz):
Are there any other
|
|
Attempt 1: Let
Consider
Choose
|
|
Also
|
|
We will use Khinchin’s inequality.