Equivalent Versions of Fourier Restriction - Fourier Restriction Theory

5 Equivalent Versions of Fourier Restriction

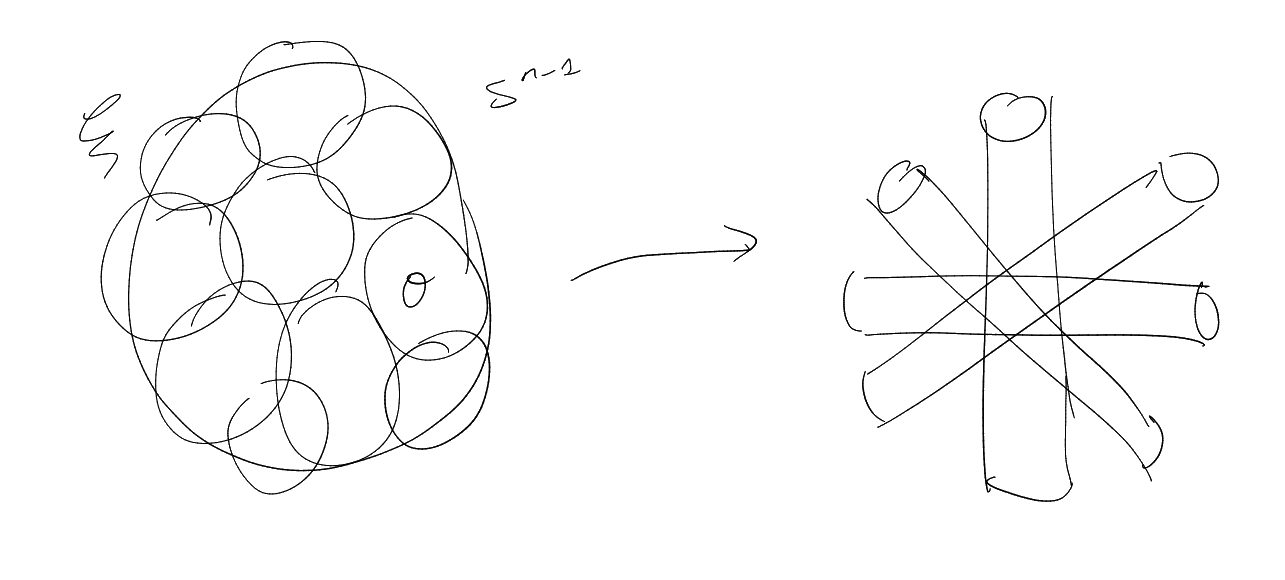
Searching for $(p, q)$ for which

∥ \hat{f} ∥_{L^{q} (S^{n - 1})} \leq C_{p, q, n} ∥ f ∥_{L^{p} (ℝ^{n})} .

(1)

$R = {[0, 1]}^{n - 1} \times {0}$
(2)

$\to \int_{B_{R^{2}}} {| \sum_{𝜃} χ_{T_{𝜃}} |}^{\frac{p}{2}}$ . “continuum incidence geometry problem”.

integral equals $= \sum_{B_{R} \subseteq B_{R^{2}}} \int_{B_{R}} {| \sum_{𝜃} χ_{T_{𝜃}} |}^{\frac{p}{2}}$ .

“fractal geometry”.

Reminder: $R_{S^{n - 1}} (p \to q)$ means

∥ \hat{f} ∥_{L^{q} (S^{n - 1})} \leq C_{p, q, n} ∥ f ∥_{L^{q} (ℝ^{n})} \forall f \in S (ℝ^{n}) .

Conjecture (Restriction Conjecture). $R_{S^{n - 1}} (p \to q)$ if and only if $n + 1 - \frac{n - 1}{q} \leq \frac{n + 1}{p}$ and $p < \frac{2 n}{n + 1}$ .

First proved for $S^{1} \subseteq ℝ^{2}$ by Fefferman (1970) and Zygmund (1974).

Special things happen in $ℝ^{2}$ , classical harmonic analysis techniques apply.

Same conjecture for $R_{P^{n - 1}} (p \to q)$ , where

P^{n - 1} = {(ξ, | ξ |^{2}) \in ℝ^{n} : | ξ | < 1} .

$∥ \hat{f} ∥_{L^{q} (P^{n - 1})}^{q} = \int_{| ξ | < 1} | \hat{f} (ξ, | ξ |^{2}) |^{q} d ξ$ .

For $n \geq 3$ , open and active!

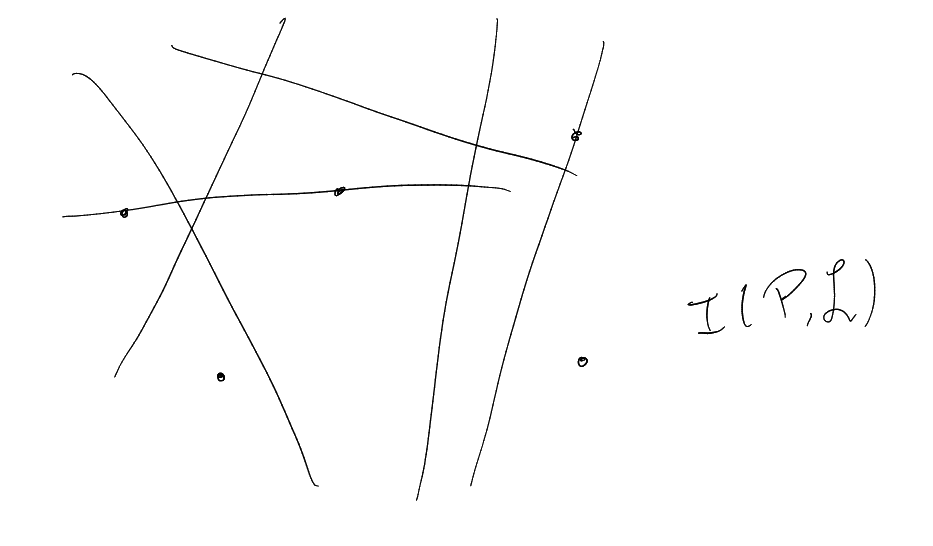
Restriction theory can be used to deduce continuum incidence geometry estimates.

Surprisingly, we can go the other way too (very recent progress, whereas the above direction has been well-known since at least the $90$ s).

Equivalent formulations of $R_{P^{n - 1}} (p \to q)$

Dual version is called “Fourier extension”:

∥ \hat{f} ∥_{L^{q} (P^{n - 1})} = \sup_{\begin{array}{c} g \in L^{q^{'}} (P^{n - 1}) \\ ∥ g ∥_{L^{q^{'}} (P^{n - 1})} = 1 \end{array}} | \int_{\begin{array}{c} | ξ | < 1 \\ ξ \in ℝ^{n - 1} \end{array}} \hat{f} (ξ, | ξ |^{2}) g (ξ) d ξ | .

( $\frac{1}{q} + \frac{1}{q^{'}} = 1$ ). The integral equals:

\begin{array}{l} \int_{| ξ | < 1} \int_{ℝ^{n}} e^{- 2 π i x \cdot (ξ, | ξ |^{2})} f (x) d x g (ξ) d ξ & = \int_{ℝ^{n}} f (x) \underset{E g (x)}{\underset{⏟}{\int_{| ξ | < 1} e^{- 2 π i x \cdot (ξ, | ξ |^{2})} g (ξ) d ξ}} d x \\ ∥ E g ∥_{L^{p^{'}} (ℝ^{n})} & \leq C_{p, q, n} ∥ g ∥_{L^{q^{'} (P^{n - 1})}} \forall S (P^{n - 1}) \end{array}

Call the last inequality $R_{P^{n - 1}}^{*} (q^{'} \to p^{'})$ .

Local, dual version: allows us to work with functions, F.T.

For any $R \geq 1$ , any $B_{R} \subseteq ℝ^{n}$ , we have

{(\int_{ℝ^{n}} | f (x) |^{p^{'}})}^{\frac{1}{p^{'}}} ≲ R^{\frac{1}{q}} {(\int | \hat{f} (ξ) |^{q^{'}} d ξ)}^{\frac{1}{q^{'}}}

for all $f \in S (ℝ^{n})$ with $supp \hat{f} \subseteq N_{R^{- 1}} (P^{n - 1})$ .

Call this $R_{P^{n - 1}}^{*, loc} (q^{'} \to p^{'})$ .

$R_{P^{n - 1}}^{*} (q^{'} \to p^{'}) ⟹ R_{p^{n - 1}}^{*, loc} (q^{'} \to p^{'})$ . First thing bounds $E g$ , while secound thing bounds $f$ when we have $supp \hat{f} \subseteq N_{R^{- 1}} (P^{n - 1})$ .

Let $f \in S (ℝ^{n})$ , $supp \hat{f} \subseteq N_{R^{- 1}} (P^{n - 1})$ . Try to express $f$ in trems of ext. op.

\begin{array}{l} f (x) & \overset{Fourier inversion}{=} \int_{ℝ^{n}} e^{2 π i x \cdot ξ} \hat{f} (ξ) d ξ \\ = \int_{ℝ^{n - 1}} \int_{ℝ} e^{2 π i x \cdot (ξ^{'}, ξ_{n})} \hat{f} (ξ^{'}, ξ_{n}) d ξ_{n} d ξ^{'} \\ = \int_{ℝ} e^{2 π x_{n} ξ_{n}} \int_{ℝ^{n - 1}} e^{2 π i x \cdot (ξ^{'}, | ξ^{'} |^{2})} \underset{= : g_{ξ_{n}} (ξ^{'})}{\underset{⏟}{\hat{f} (ξ^{'}, | ξ^{'} |^{2} + ξ_{n})}} d ξ^{'} d ξ_{n} & ξ_{n} \mapsto ξ_{n} + | ξ^{'} |^{2} \\ = \int_{[- R^{- 1}, R^{- 1}]} e^{2 π x_{n} ξ_{n}} \underset{E g_{ξ_{n}} (x)}{\underset{⏟}{\int_{| ξ^{'} | < 1} e^{2 π i x \cdot (ξ^{'}, | ξ^{'} |^{2})} \underset{= : g_{ξ_{n}} (ξ^{'})}{\underset{⏟}{\hat{f} (ξ^{'}, | ξ^{'} |^{2} + ξ_{n})}} d ξ^{'}}} d ξ_{n} \\ \int_{ℝ^{n}} | f (x) |^{p^{'}} d x & = \int_{ℝ^{n}} {| \int_{I_{R^{-} 1}} e^{2 π i x_{n} ξ_{n}} E g_{ξ_{n}} (x) d ξ_{n} |}^{p^{'}} d x & R^{*} bounds \int | E g |^{p^{'}} \\ \overset{Hölders’s}{\leq} \int_{ℝ^{n}} | I_{R^{-} 1} |^{p^{'} - 1} \int_{I_{R^{- 1}}} | E g_{ξ_{n} (x)} |^{p^{'}} d ξ_{n} d x \\ ≲ \underset{(*)}{\underset{⏟}{{(R^{- 1})}^{p^{'} - 1} \int_{I_{R^{- 1}}} {(\int_{| ξ^{'} | < 1} | g_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'})}^{\frac{p^{'}}{q^{'}}} d ξ_{n}}} & using R_{P^{n - 1}}^{*} (q^{'} \to p^{'}) \end{array}

Goal is to bound this last expression by

R^{- \frac{p^{'}}{q}} \underset{∥ \hat{f} ∥_{L^{q^{'}} (N_{R^{- 1}} (P^{n - 1}))}}{\underset{⏟}{\int_{I_{R^{- 1}}} \int_{| ξ^{'} | < 1} | g_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'} d ξ_{n}}}

Lucy case: $\frac{p^{'}}{q^{'}} < 1$ , i.e. $1 < \frac{q^{'}}{p^{'}}$ . Then

(*) \leq R^{- p^{'} + 1} \int_{I_{R^{- 1}}}

\int_{A} h \overset{Hölder’s}{\leq} | A |^{1 - \frac{1}{s}} {(\int_{A} h^{s})}^{\frac{1}{s}},

$s \geq 1$ (actually $\approx$ since $h$ approximately constant on $A$ )

$R_{P^{n - 1}}^{*} (q^{'} \to p^{'}) ⟹ R_{P^{n - 1}}^{*, loc} (q^{'} \to p^{'})$ continued.

Spatial: $x, f (x), E g (x)$

\int_{ℝ^{n}} | f (x) |^{p^{'}} d x

\int_{ℝ^{n}} | E g (x) |^{p^{'}} d x

Frequency $ξ$ :

$g (ξ^{'}, | ξ^{'} |^{2}) = g (ξ^{'})$ , $supp \hat{f} \subseteq N_{R^{- 1}} (P^{n - 1})$ ,

\int_{N_{R^{- 1}} (P^{n - 1})} | \hat{f} (ξ) |^{q^{'}} d ξ

\int_{| ξ^{'} | < 1} | g (ξ^{'}) |^{q^{'}} d ξ^{'} .

\int_{B_{R}} | f (x) |^{p^{'}} d x \leq \dots \overset{(*)}{\leq} R^{- (p^{'} - 1)} \int_{I_{R^{- 1}}} {(\int_{| ξ^{'} | < 1} | g_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'})}^{\frac{p^{'}}{q^{'}}} d ξ_{n} .

Aiming for

(*) \leq R^{- \frac{p^{'}}{q}} {(\int_{I_{R^{- 1}}} \int_{| ξ^{'} | < 1} | g_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'})}^{\frac{p^{'}}{q^{'}}} .

Lucky case: $\frac{p^{'}}{q^{'}} \leq 1$ .

(*) \overset{Hölder’s inequality in ξ_{n}}{\leq} R^{- (p^{'} - 1)} | I_{R^{- 1}} |^{1 - \frac{p^{'}}{q^{'}}} {(\int_{I_{R^{- 1}}} \int_{| ξ^{'} | < 1} | g_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'} d ξ_{n})}^{\frac{p^{'}}{q^{'}}}

$R^{- (p^{'} - 1)} {(R^{- 1})}^{1 - \frac{p^{'}}{q^{'}}} = R^{- \frac{p^{'}}{q}}$ ( $1 = \frac{1}{q} + \frac{1}{q^{'}}$ )

Unlucky case: $\frac{p^{'}}{q^{'}} > 1$ . Hölder’s inequality goes in the wrong direction:

\int_{A} h \leq | A |^{1 - \frac{1}{s}} {(\int_{A} h^{s})}^{\frac{1}{s}}

for all $s \geq 1$ , $h \geq 0$ .

This is equality if $h$ is constant on $A$ .

PAUSE THIS.

Useful Harmonic Analysis tool

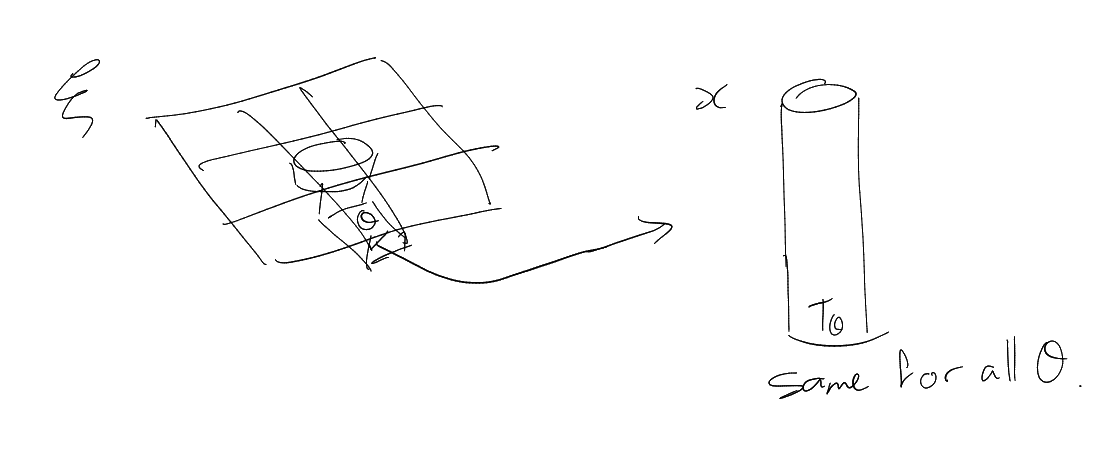
The locally constant property.

Convolution: Let $f, g \in S (ℝ^{n})$ . Define $f * g \in S (ℝ^{n})$ by

f * g (x) = \int_{ℝ^{n}} f (x - y) g (y) d y = \int_{ℝ^{n}} f (Y) g (x - y) d y .

See Young’s convolution:

∥ f * g ∥_{L^{r}} \leq ∥ f ∥_{p} ∥ g ∥_{q}

when $1 + \frac{1}{r} = \frac{1}{p} + \frac{1}{q}$ .

Example. $g = χ_{B}$ ( $B$ is the unit ball in $ℝ^{n}$ ),

f * χ_{B} (x) = \int_{y \in B} f (x - y) d y

RHS is “average value of $f$ on $B (x)$ ”.

“ $f * χ_{B}$ is approximately constant on balls of radius $\sim 1$ ”.

Support property: $supp f = A$ , $supp g = B$ , $supp f * g \subseteq A + B = {a + b : a \in A, b \in B}$ .

Convolution and Fourier Transform: $\hat{f g} = \hat{f} * \hat{g}$ .

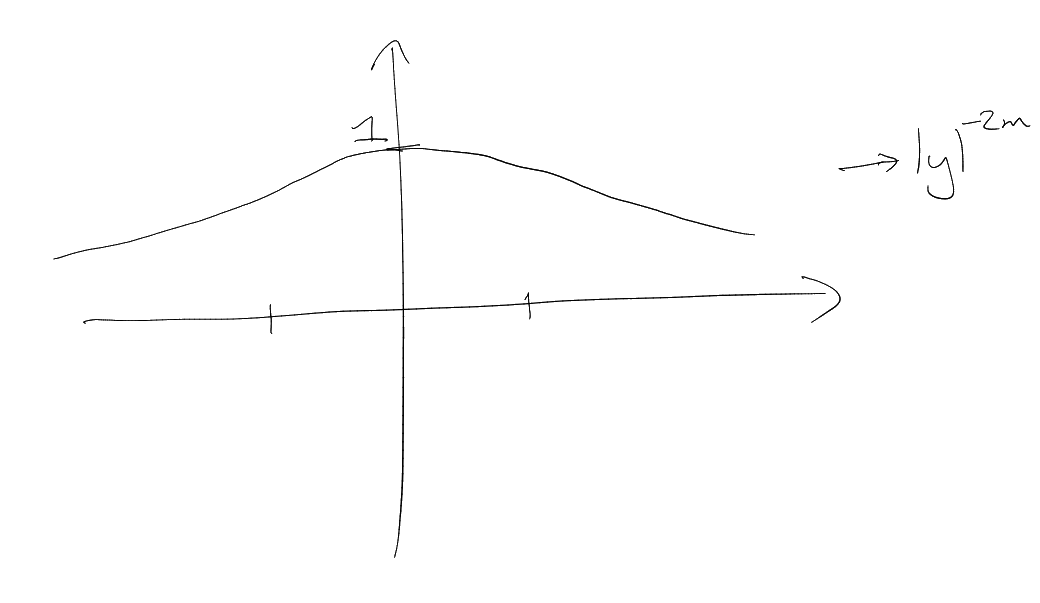
Locally constant property: Let $f \in S (ℝ^{n})$ , $supp \hat{f} \subseteq B_{1} (0)$ . Then for any unit ball $B^{'} \subseteq ℝ^{n}$ and any $x \in B^{'}$ ,

∥ f ∥_{L^{\infty} (B^{'})} ≲_{m} \int_{ℝ^{n}} | f | (x - y) \frac{1}{{(1 + | y |^{2})}^{m}} d y .

$\frac{1}{{(1 + | y |^{2})}^{m}}$ is $\sim 1$ on $| y | ≲ 1$ .

Digesting $f \geq 0$ .

For any unit interval $I^{'} \subseteq ℝ^{n}$ , $x \in I^{'}$ ,

∥ f ∥_{L^{\infty} (I^{'})} \leq \int_{(- \frac{1}{2}, \frac{1}{2})} f (x - y) d y .

Suppose this:

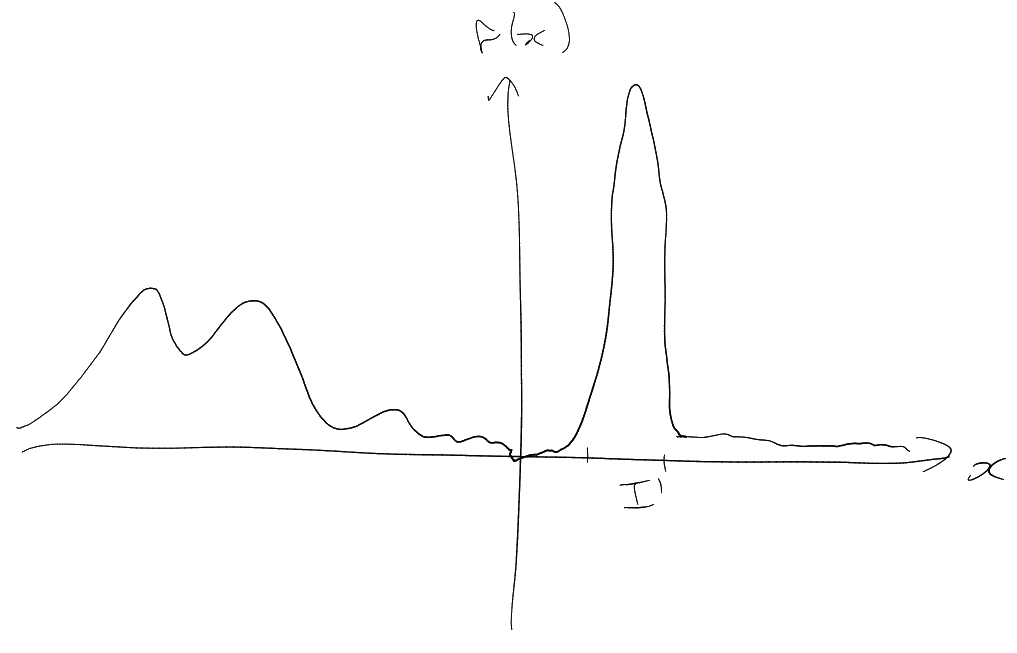
LHS has to be constant.

∥ f ∥_{L^{\infty} (I^{'})} ≲ \int_{(- \frac{1}{2}, \frac{1}{2})} f (x - y) d y .

∥ f ∥_{L^{\infty} (I^{'})} \overset{(*)}{≲} \int_{(- \frac{1}{2}, \frac{1}{2})} f (x - y) d y

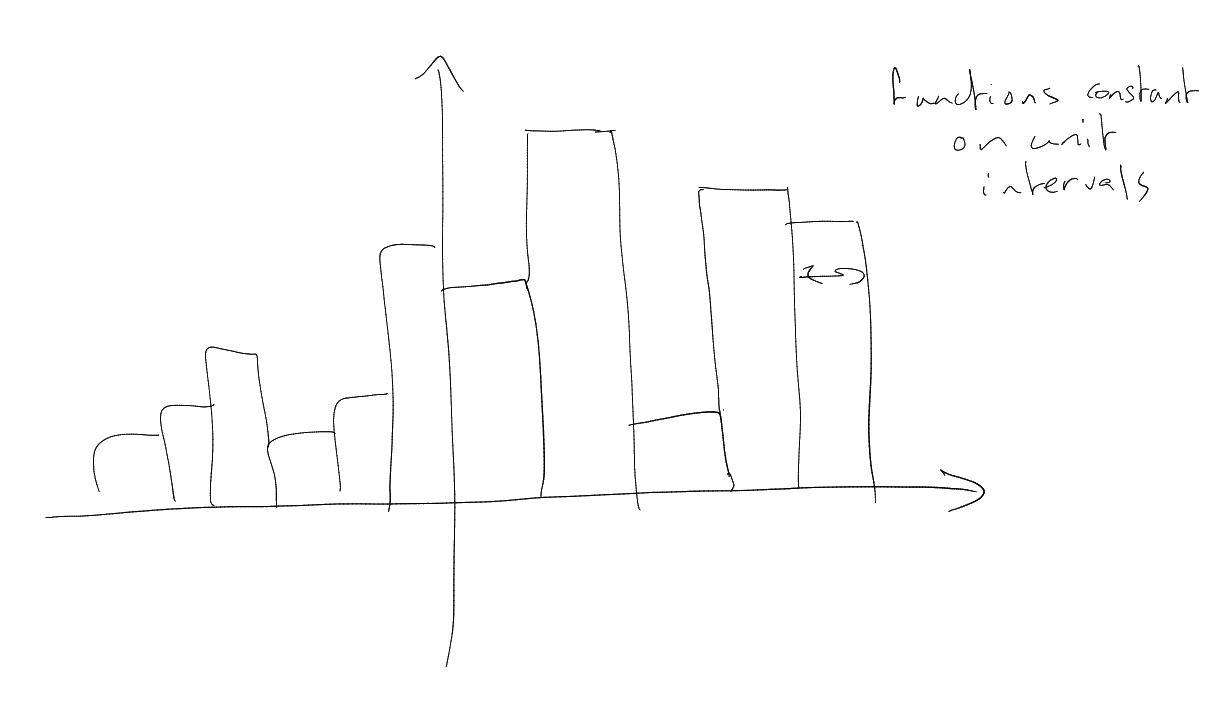
Lemma (Locally constant property). Assuming that:

$f \in S (ℝ^{n})$
$supp \hat{f} \subseteq B_{1} (0)$

Then for any unit ball

B^{1} \subseteq ℝ^{n}

and any

x \in B^{'}

∥ f ∥_{L^{\infty} (B^{'})} ≲_{m} \int_{ℝ^{n}} | f | (x - y) \frac{1}{{(1 + | y |^{2})}^{m}} d y .

The proof of this fact is more important than the statement – we will be using the strategy in future.

Proof of locally constant property. Let $f \in S (ℝ^{n})$ , $supp \hat{f} \subseteq B_{1} (0)$ . Let $φ \in C_{c}^{\infty} (ℝ^{n})$ such that $φ \equiv 1$ on $B_{1} (0)$ , $supp φ \subseteq B_{2} (0)$ .

By Fourier inversion:

f = ˇ (\hat{f}) = ˇ (\hat{f} φ) = ˇ (\hat{f}) * ˇ φ = f * ˇ φ .

Let $x_{0}, x \in B^{1}$ (unit ball in $ℝ^{n}$ ).

\begin{array}{l} | f (x_{0}) | & = | \int f (x_{0} - y) ˇ φ (y) d y | \\ \leq \int | f | (x_{0} - y) | ˇ φ (y) | d y \\ = \int | f | (x - y) | ˇ φ | (y - (y - x_{0})) d y \\ ≲_{m} \int | f | (x - y) \frac{1}{{(1 + | y - \underset{| x - x_{0} | < 1}{\underset{⏟}{(x - x_{0})}} |^{2})}^{m}} d y \\ ≲_{m} \int | f | (x - y) \frac{1}{{(1 + | y |^{2})}^{m}} □ \end{array}

Returning to $R_{P^{n - 1}}^{} (q^{'} \to p^{'}) ⟹ R_{P^{n - 1}}^{, loc} (q^{'} \to p^{'})$

\int_{B_{R}} | f (x) |^{p^{'}} d x ≲ \int_{B_{R}} | f φ_{B_{R}} |^{p^{'}} d x

where $φ_{B_{R}} \in S (ℝ^{n})$ satisfies $φ_{B_{R}} \sim 1$ on $B_{R}$ , $supp \hat{φ_{B_{R}}} \subseteq B_{R^{- 1}} (0)$ .

\hat{f φ_{B_{R}}} = \underset{R^{- 1} neighbourhood of P^{n - 1}}{\underset{⏟}{\hat{f}}} * \underset{R^{- 1} ball}{\underset{⏟}{\hat{φ_{B_{R}}}}} (ξ)

supported in $2 R^{- 1}$ -neighbourhood of $P^{n - 1}$ .

Repeat steps of proof:

\begin{array}{l} \int_{B_{R}} | f (x) |^{p^{'}} d x & ≲ R^{- (p^{'} - 1)} \int_{I_{R^{- 1}}} {(\int_{| ξ^{'} | < 1} | h_{ξ_{n}} (ξ^{'}) |^{q^{'}} d ξ^{'})}^{\frac{p^{'}}{q^{'}}} d ξ_{n} \end{array}

h (ξ_{n}) = \hat{f} * \hat{φ_{B_{R}}} (ξ^{'}, | ξ^{'} |^{2} + ξ_{n})

[next] [prev] [prev-tail] [front] [up]

5 Equivalent Versions of Fourier Restriction

Equivalent formulations of RPn−1(p→q)

Useful Harmonic Analysis tool

Returning to RPn−1∗(q′→p′)⟹RPn−1∗,loc(q′→p′)

Equivalent formulations of $R_{P^{n - 1}} (p \to q)$

Returning to $R_{P^{n - 1}}^{} (q^{'} \to p^{'}) ⟹ R_{P^{n - 1}}^{, loc} (q^{'} \to p^{'})$