9 Dedekind domains
Definition 9.1 (Dedekind domain).
A Dedekind domain is a ring
-
(i)
-
(ii)
- (iii) Every non-zero prime ideal is maximal.
Example.
-
The ring of integers in a number field is a Dedekind domain.
-
Any PID (hence a discrete valuation ring) is a Dedekind domain.
Theorem 9.2.
A ring
Proof.
Suppose not. Since
Let
-
-
-
Proof.
Let
|
|
for some
Then in
|
|
Multiply by
|
|
Hence
Proof of Theorem 9.2.
-
-
Step 1:
Let
Set
Step 2:
Let
Let
|
|
If
Example.
-
-
Facts: (not proved in this course, but can be found in a typical course / textbook on commutative algebra)
-
-
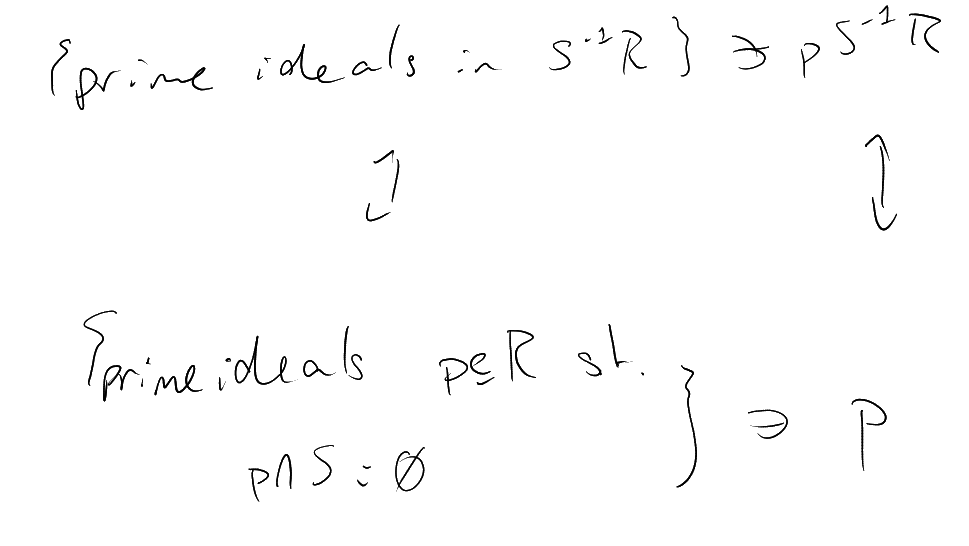
Corollary 9.5.
Let
Proof.
By properties of localisation,
It suffices to show
Let
|
|
with
Definition 9.6 (Valuation on a Dedekind domain).
If
Example.
-
-
|
|
(with
Remark. Clear for PIDs (PID implies UFD).
Proof (Sketch). We quote the following properties of localisation:
-
(i)
-
(ii)
If
Let
Let
Corollary 9.5 gives
For uniqueness, if